Effect size for RM Bayesian Anova

johan_achard

Hello everyone,

I have a question about the effect size for the Bayesian RM ANOVA. Should we report an effect size for B-ANOVA as in frequentist?

On the JASP forum, it is suggested that the R^2 value for Bayesian ANOVA can be similar to the effect size. R2 is generally used for regression, and since Bayesian RM ANOVA is based on the Bayesian mixed model, I think I'm familiar with the concept.

In another discussion, I read that vandenman explained "The only available effect size measure for the Bayesian ANOVAs is R^2. This is computed for each model and you can obtain a model-averaged R^2. [...].", and another "The Bayesian R-squared is derived from Gelman's unpublished manuscript. So R2 is based on the variance of estimated prediction errors. The prediction errors come from the model averaged posterior, so it's not a point estimate of the best model."

Does the latest version of JASP allow R2 to be calculated for all predictors in the Bayesian model? And in concrete terms, what do you recommend reporting the Bayesian analysis and if a reviewer asks for the effect size in addition?

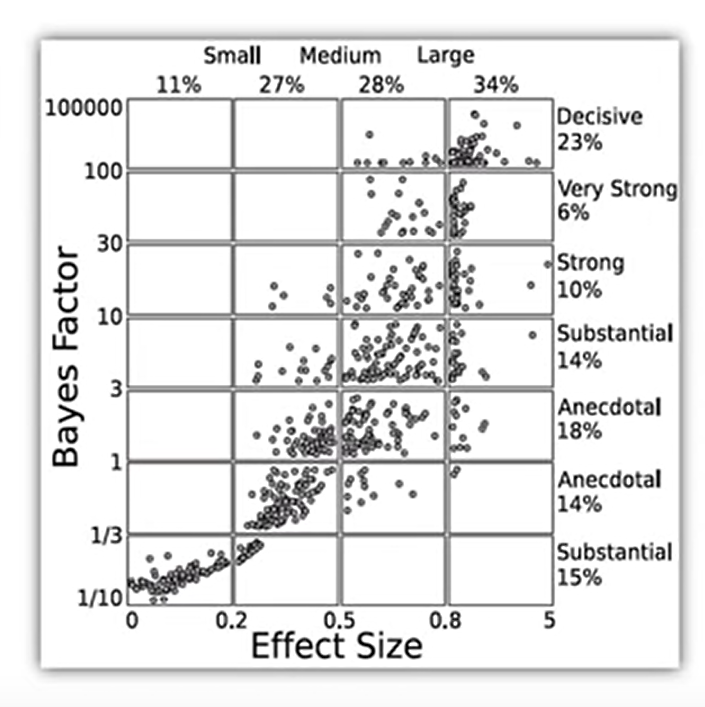
Also, I see the following picture here apparently published in Wetzels et al (2011), Perspectives on Psychological Science, 6, 291-298, https://doi.org/10.1177/1745691611406923, but I don't understand... What do you think ?

Thanks for your help!

Johan

Discussions on the JASP forum for those interested in these questions:

- https://forum.cogsci.nl/discussion/8070/how-to-calculate-effect-size-in-bayesian-repeated-measures-anova

- https://forum.cogsci.nl/discussion/7316/effect-sizes-for-bayesian-mixed-anova-and-mann-whitney-u-test

- https://forum.cogsci.nl/discussion/6139/jasp-bayesian-ancova-model-averaged-r2

EJ

Dear Johan,

The figure is not so relevant since it maps the empirical function from effect size to Bayes factors. For your effect size question I've notified the team...

Cheers,

E.J.